Understanding dense hydrogen at planetary conditions

Materials at high pressures and temperatures are of great interest for planetary science and astrophysics, warm dense-matter physics and inertial confinement fusion research. Planetary structure models rely on an understanding of the behaviour of elements and their mixtures under conditions that do not exist on Earth; at the same time, planets serve as natural laboratories for studying materials at extreme conditions. The topic of dense hydrogen is timely given the recent accurate measurements of the gravitational fields of Jupiter and Saturn, the current and upcoming progress in shock experiments, and the advances in numerical simulations of materials at high pressure. In this Review we discuss the connection between modelling planetary interiors and the high-pressure physics of hydrogen and helium. We summarize key experiments and theoretical approaches for determining the equation of state and phase diagram of hydrogen and helium. We relate this to current knowledge of the internal structures of Jupiter and Saturn, and discuss the importance of high-pressure physics to their characterization.

Key points

This is a preview of subscription content, access via your institution

Access options

Access Nature and 54 other Nature Portfolio journals

Get Nature+, our best-value online-access subscription

cancel any time

Subscribe to this journal

Receive 12 digital issues and online access to articles

111,02 € per year

only 9,25 € per issue

Buy this article

Prices may be subject to local taxes which are calculated during checkout

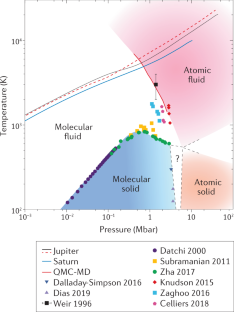
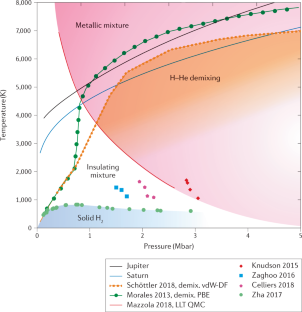
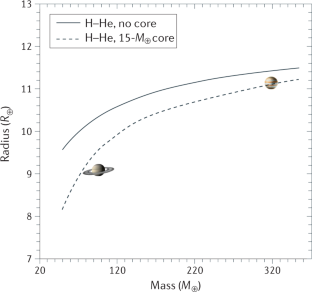
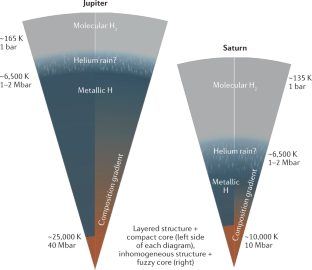
Similar content being viewed by others

Evidence of hydrogen−helium immiscibility at Jupiter-interior conditions

Article 26 May 2021

Structure and density of silicon carbide to 1.5 TPa and implications for extrasolar planets

Article Open access 27 April 2022

Implications of the iron oxide phase transition on the interiors of rocky exoplanets

Article 11 February 2021

References

  1. McMahon, J. M., Morales, M. A., Pierleoni, C. & Ceperley, D. M. The properties of hydrogen and helium under extreme conditions. Rev. Mod. Phys.84, 1607–1653 (2012). ADSGoogle Scholar
  2. Wigner, E. & Huntington, H. B. On the possibility of a metallic modification of hydrogen. J. Chem. Phys.3, 764–770 (1935). ADSGoogle Scholar
  3. Helled, R., Anderson, J. D., Podolak, M. & Schubert, G. Interior models of Uranus and Neptune. Astrophys. J.726, 15 (2011). ADSGoogle Scholar
  4. Ashcroft, N. W. Metallic hydrogen: a high-temperature superconductor? Phys. Rev. Lett.21, 1748–1749 (1968). ADSGoogle Scholar
  5. Babaev, E., Sudbø, A. & Ashcroft, N. A superconductor to superfluid phase transition in liquid metallic hydrogen. Nature431, 666 (2004). ADSGoogle Scholar
  6. Goncharov, A. Phase diagram of hydrogen at extreme pressures and temperatures; updated through 2019 (Review article). Low Temp. Phys.46, 97–103 (2020). ADSGoogle Scholar
  7. Weir, S., Mitchell, A. & Nellis, W. Metallization of fluid molecular hydrogen at 140 GPa (1.4 Mbar). Phys. Rev. Lett.76, 1860 (1996). ADSGoogle Scholar
  8. Goncharov, A. F., Mazin, I. I., Eggert, J. H., Hemley, R. J. & Mao, H.-k Invariant points and phase transitions in deuterium at megabar pressures. Phys. Rev. Lett.75, 2514–2517 (1995). ADSGoogle Scholar
  9. Dias, R. P. & Silvera, I. F. Observation of the Wigner–Huntington transition to metallic hydrogen.Science355, 715–718 (2017). ADSGoogle Scholar
  10. Datchi, F., Loubeyre, P. & LeToullec, R. Extended and accurate determination of the melting curves of argon, helium, ice (H2O), and hydrogen (H2). Phys. Rev. B61, 6535–6546 (2000). ADSGoogle Scholar
  11. Deemyad, S. & Silvera, I. F. Melting line of hydrogen at high pressures. Phys. Rev. Lett.100, 155701 (2008). ADSGoogle Scholar
  12. Dzyabura, V., Zaghoo, M. & Silvera, I. F. Evidence of a liquid–liquid phase transition in hot dense hydrogen. Proc. Natl Acad. Sci. USA110, 8040–8044 (2013). ADSGoogle Scholar
  13. Zaghoo, M., Salamat, A. & Silvera, I. F. Evidence of a first-order phase transition to metallic hydrogen. Phys. Rev. B93, 155128 (2016). ADSGoogle Scholar
  14. Zaghoo, M. & Silvera, I. F. Conductivity and dissociation in liquid metallic hydrogen and implications for planetary interiors. Proc. Natl Acad. Sci. USA114, 11873–11877 (2017). ADSGoogle Scholar
  15. Dubrovinsky, L. et al. The most incompressible metal osmium at static pressures above 750 gigapascals. Nature525, 226–229 (2015). ADSGoogle Scholar
  16. Loubeyre, P., Occelli, F. & Dumas, P. Synchrotron infrared spectroscopic evidence of the probable transition to metal hydrogen. Nature577, 631–635 (2020). ADSGoogle Scholar
  17. Goncharov, A. F. & Struzhkin, V. V. Comment on ‘‘Observation of the Wigner–Huntington transition to metallic hydrogen’’. Science357, eaam9736 (2017). Google Scholar
  18. Liu, X.-D. & Dalladay-Simpson, P. & Howie, R. T. & Li, B. & Gregoryanz, E. Comment on ‘‘Observation of the Wigner–Huntington transition to metallic hydrogen”. Science357, eaan2286 (2017). Google Scholar
  19. Loubeyre, P., Occelli, F. & Dumas, P. Comment on: ‘‘Observation of the Wigner–Huntington transition to metallic hydrogen’’. Preprint at http://arxiv.org/abs/1702.07192 (2017).
  20. Eremets, M. & Drozdov, A. Comments on the claimed observation of the Wigner–Huntington transition to metallic hydrogen. Preprint at https://arxiv.org/abs/1702.05125 (2017).
  21. Geng, H. Y. Public debate on metallic hydrogen to boost high pressure research. Matter Radiat. Extremes2, 275 (2018). Google Scholar
  22. Silvera, I. & Dias, R. Response to comment on ‘‘Observation of the Wigner–Huntington transition to metallic hydrogen”. Science357, eaan1215 (2017). Google Scholar
  23. Silvera, I. & Dias, R. Response to critiques on observation of the Wigner–Huntington transition to metallic hydrogen. Preprint at http://arxiv.org/abs/1703.0306 (2017).
  24. Howie, R. T., Dalladay-Simpson, P. & Gregoryanz, E. Raman spectroscopy of hot hydrogen above 200 GPa. Nat. Mater.14, 495–499 (2015). ADSGoogle Scholar
  25. Dalladay-Simpson, P., Howie, R. T. & Gregoryanz, E. Evidence for a new phase of dense hydrogen above 325 gigapascals. Nature529, 63–67 (2016). ADSGoogle Scholar
  26. Eremets, M., Troyan, I. & Drozdov, A. Low temperature phase diagram of hydrogen at pressures up to 380 GPa. A possible metallic phase at 360 GPa and 200 K. Preprint at http://arxiv.org/abs/1601.04479 (2016).
  27. Dias, R. P., Noked, O. & Silvera, I. F. Quantum phase transition in solid hydrogen at high pressure. Phys. Rev. B100, 184112 (2019). ADSGoogle Scholar
  28. Eremets, M. I., Drozdov, A. P., Kong, P. & Wang, H. Semimetallic molecular hydrogen at pressure above 350 GPa. Nat. Phys.15, 1246–1249 (2019). Google Scholar
  29. Gregoryanz, E. et al. Everything you always wanted to know about metallic hydrogen but were afraid to ask. Matter Radiat. Extremes5, 038101 (2020). Google Scholar
  30. Dias, R. P. & Silvera, I. F. Observation of the Wigner–Huntington transition to metallic hydrogen. Science355, 715–718 (2017). ADSGoogle Scholar
  31. Nellis, W. Ultracondensed Matter by Dynamic Compression (Cambridge Univ. Press, 2017).
  32. Nellis, W. J. Dense quantum hydrogen. Low Temp. Phys.45, 294–296 (2019). ADSGoogle Scholar
  33. Knudson, M. et al. Direct observation of an abrupt insulator-to-metal transition in dense liquid deuterium. Science348, 1455–1460 (2015). ADSGoogle Scholar
  34. Celliers, P. M. et al. Insulator–metal transition in dense fluid deuterium. Science361, 677–682 (2018). ADSGoogle Scholar
  35. Mochalov, M. A. et al. Quasi-isentropic compressibility of deuterium at a pressure of ~12 TPa. JETP Lett.107, 168–174 (2018). ADSGoogle Scholar
  36. Brygoo, S. et al. Analysis of laser shock experiments on precompressed samples using a quartz reference and application to warm dense hydrogen and helium. J. Appl. Phys.118, 195901 (2015). ADSGoogle Scholar
  37. Miller, J. E. et al. Streaked optical pyrometer system for laser-driven shock-wave experiments on omega. Rev. Sci. Instrum.78, 034903 (2007). ADSGoogle Scholar
  38. Knudson, M. D. & Desjarlais, M. P. High-precision shock wave measurements of deuterium: evaluation of exchange-correlation functionals at the molecular-to-atomic transition. Phys. Rev. Lett.118, 035501 (2017). ADSGoogle Scholar
  39. Knudson, M. D. et al. Probing the interiors of the ice giants: shock compression of water to 700 GPa and 3.8 g/cm 3 . Phys. Rev. Lett.108, 091102 (2012). ADSGoogle Scholar
  40. Millot, M. et al. Nanosecond X-ray diffraction of shock-compressed superionic water ice. Nature569, 251 (2019). ADSGoogle Scholar
  41. Eremets, M. I. & Trojan, I. Evidence of maximum in the melting curve of hydrogen at megabar pressures. JETP Lett.89, 174–179 (2009). ADSGoogle Scholar
  42. Subramanian, N., Goncharov, A. F., Struzhkin, V. V., Somayazulu, M. & Hemley, R. J. Bonding changes in hot fluid hydrogen at megabar pressures. Proc. Natl Acad. Sci. USA108, 6014–6019 (2011). ADSGoogle Scholar
  43. Zha, C.-s, Liu, H., Tse, J. S. & Hemley, R. J. Melting and high P−T transitions of hydrogen up to 300 GPa. Phys. Rev. Lett.119, 075302 (2017). Google Scholar
  44. Zha, C.-S., Liu, Z., Ahart, M., Boehler, R. & Hemley, R. J. High-pressure measurements of hydrogen phase IV using synchrotron infrared spectroscopy. Phys. Rev. Lett.110, 217402 (2013). ADSGoogle Scholar
  45. Mott, N. F. The transition to the metallic state. Phil. Mag.6, 287–309 (1961). ADSGoogle Scholar
  46. Ohta, K. et al. Phase boundary of hot dense fluid hydrogen. Sci. Rep.5, 16560 (2015). ADSGoogle Scholar
  47. Ross, M., Ree, F. & Young, D. The equation of state of molecular hydrogen at very high density. J. Chem. Phys.79, 1487–1494 (1983). ADSGoogle Scholar
  48. Saumon, D., Chabrier, G. & Van Horn, H. An equation of state for low-mass stars and giant planets. Astrophys. J. Suppl. Ser.99, 713 (1995). ADSGoogle Scholar
  49. Chabrier, G., Mazevet, S. & Soubiran, F. A new equation of state for dense hydrogen–helium mixtures. Astrophys. J.872, 51 (2019). ADSGoogle Scholar
  50. Ross, M. Linear-mixing model for shock-compressed liquid deuterium. Phys. Rev. B58, 669–677 (1998). ADSGoogle Scholar
  51. Kerley, G. I. Equations of state for hydrogen and deuterium. Sandia National Laboratories report SAND 2003–3613 (SAND, 2003).
  52. Caillabet, L., Mazevet, S. & Loubeyre, P. Multiphase equation of state of hydrogen from ab initio calculations in the range 0.2 to 5 g/cc up to 10 eV. Phys. Rev. B83, 094101 (2011). ADSGoogle Scholar
  53. Militzer, B. & Hubbard, W. B. Ab initio equation of state for hydrogen–helium mixtures with recalibration of the giant-planet mass–radius relation. Astrophys. J.774, 148 (2013). ADSGoogle Scholar
  54. Militzer, B. Equation of state calculations of hydrogen–helium mixtures in solar and extrasolar giant planets. Phys. Rev. B87, 014202 (2013). ADSGoogle Scholar
  55. Becker, A. et al. Ab initio equations of state for hydrogen (H-REOS. 3) and helium (He-REOS. 3) and their implications for the interior of brown dwarfs. Astrophys. J. Suppl. Ser.215, 21 (2014). ADSGoogle Scholar
  56. Brush, S., Sahlin, H. & Teller, E. Monte Carlo study of a one-component plasma. I. J. Chem. Phys.45, 2102–2118 (1966). ADSGoogle Scholar
  57. Morales, M. A., McMahon, J. M., Pierleoni, C. & Ceperley, D. M. Nuclear quantum effects and nonlocal exchange-correlation functionals applied to liquid hydrogen at high pressure. Phys. Rev. Lett.110, 065702 (2013). ADSGoogle Scholar
  58. Allen, M. P. & Tildesley, D. J. Computer Simulation of Liquids (Clarendon, 1987).
  59. Pierleoni, C., Ceperley, D. M. & Holzmann, M. Coupled electron–ion Monte Carlo calculations of dense metallic hydrogen. Phys. Rev. Lett.93, 146402 (2004). ADSGoogle Scholar
  60. Payne, M. C., Teter, M. P., Allan, D. C., Arias, T. A. & Joannopoulos, J. D. Iterative minimization techniques for ab initio total-energy calculations: molecular dynamics and conjugate gradients. Rev. Mod. Phys.64, 1045–1097 (1992). ADSGoogle Scholar
  61. Alavi, S.Book review: Ab initio Molecular Dynamics. Basic Theory and Advanced Methods. By Dominik Marx and Jürg Hutter. Angew. Chem. Int. Ed.48, 9404–9405 (2009). Google Scholar
  62. Andersen, H. C. Molecular dynamics simulations at constant pressure and/or temperature. J. Chem. Phys.72, 2384–2393 (1980). ADSGoogle Scholar
  63. Martyna, G. J., Tobias, D. J. & Klein, M. L. Constant pressure molecular dynamics algorithms. J. Chem. Phys.101, 4177–4189 (1994). ADSGoogle Scholar
  64. Bussi, G., Donadio, D. & Parrinello, M. Canonical sampling through velocity rescaling. J. Chem. Phys.126, 014101 (2007). ADSGoogle Scholar
  65. Ceperley, D. M. & Alder, B. J. Ground state of solid hydrogen at high pressures. Phys. Rev. B36, 2092–2106 (1987). ADSGoogle Scholar
  66. Cao, J. & Voth, G. A. The formulation of quantum statistical mechanics based on the Feynman path centroid density. I. Equilibrium properties. J. Chem. Phys.100, 5093–5105 (1994). ADSGoogle Scholar
  67. Ceperley, D. M. Path integrals in the theory of condensed helium. Rev. Mod. Phys.67, 279–355 (1995). ADSGoogle Scholar
  68. Pierleoni, C., Morales, M. A., Rillo, G., Holzmann, M. & Ceperley, D. M. Liquid–liquid phase transition in hydrogen by coupled electron–ion Monte Carlo simulations. Proc. Natl Acad. Sci. USA113, 4953–4957 (2016). ADSGoogle Scholar
  69. Car, R. & Parrinello, M. Unified approach for molecular dynamics and density-functional theory. Phys. Rev. Lett.55, 2471–2474 (1985). ADSGoogle Scholar
  70. Hohenberg, P. & Kohn, W. Inhomogeneous electron gas. Phys. Rev.136, B864–B871 (1964). ADSMathSciNetGoogle Scholar
  71. Kohn, W. & Sham, L. J. Self-consistent equations including exchange and correlation effects. Phys. Rev.140, A1133–A1138 (1965). ADSMathSciNetGoogle Scholar
  72. Burke, K. Perspective on density functional theory. J. Chem. Phys.136, 150901 (2012). ADSGoogle Scholar
  73. Cohen, A. J., Mori-Sánchez, P. & Yang, W. Challenges for density functional theory. Chem. Rev.112, 289–320 (2011). Google Scholar
  74. Perdew, J. P., Burke, K. & Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett.77, 3865–3868 (1996). ADSGoogle Scholar
  75. Scandolo, S. Liquid–liquid phase transition in compressed hydrogen from first-principles simulations. Proc. Natl Acad. Sci. USA100, 3051–3053 (2003). ADSGoogle Scholar
  76. Lorenzen, W., Holst, B. & Redmer, R. First-order liquid–liquid phase transition in dense hydrogen. Phys. Rev. B82, 195107 (2010). ADSGoogle Scholar
  77. Vorberger, J., Tamblyn, I., Militzer, B. & Bonev, S. A. Hydrogen–helium mixtures in the interiors of giant planets. Phys. Rev. B75, 024206 (2007). ADSGoogle Scholar
  78. Tamblyn, I. & Bonev, S. A. Structure and phase boundaries of compressed liquid hydrogen. Phys. Rev. Lett.104, 065702 (2010). ADSGoogle Scholar
  79. Morales, M. A., Pierleoni, C., Schwegler, E. & Ceperley, D. M. Evidence for a first-order liquid–liquid transition in high-pressure hydrogen from ab initio simulations. Proc. Natl Acad. Sci. USA107, 12799–12803 (2010). ADSGoogle Scholar
  80. Bonev, S., Schwegler, E., Galli, G. & Ogitsu, T. A quantum fluid of metallic hydrogen suggested by first-principles calculations. Nature431, 669 (2004). ADSGoogle Scholar
  81. Pickard, C. J. & Needs, R. J. Structure of phase III of solid hydrogen. Nat. Phys.3, 473 (2007). Google Scholar
  82. Liu, H., Zhu, L., Cui, W. & Ma, Y. Room-temperature structures of solid hydrogen at high pressures. J. Chem. Phys.137, 074501 (2012). ADSGoogle Scholar
  83. Magda˘u, I. B. & Ackland, G. J. Identification of high-pressure phases III and IV in hydrogen: simulating Raman spectra using molecular dynamics. Phys. Rev. B87, 174110 (2013). ADSGoogle Scholar
  84. Pickard, C. J., Martinez-Canales, M. & Needs, R. J. Density functional theory study of phase IV of solid hydrogen. Phys. Rev. B85, 214114 (2012). ADSGoogle Scholar
  85. Naumov, I. I., Hemley, R. J., Hoffmann, R. & Ashcroft, N. Chemical bonding in hydrogen and lithium under pressure. J. Chem. Phys.143, 064702 (2015). ADSGoogle Scholar
  86. Monserrat, B. et al. Structure and metallicity of phase V of hydrogen. Phys. Rev. Lett.120, 255701 (2018). ADSGoogle Scholar
  87. Morales, M. A. et al. Phase separation in hydrogen–helium mixtures at Mbar pressures. Proc. Natl Acad. Sci. USA106, 1324–1329 (2009). ADSGoogle Scholar
  88. Lorenzen, W., Holst, B. & Redmer, R. Demixing of hydrogen and helium at megabar pressures. Phys. Rev. Lett.102, 115701 (2009). ADSGoogle Scholar
  89. Becke, A. D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A38, 3098–3100 (1988). ADSGoogle Scholar
  90. Monserrat, B., Ashbrook, S. E. & Pickard, C. J. Nuclear magnetic resonance spectroscopy as a dynamical structural probe of hydrogen under high pressure. Phys. Rev. Lett.122, 135501 (2019). ADSGoogle Scholar
  91. Monserrat, B., Needs, R. J., Gregoryanz, E. & Pickard, C. J. Hexagonal structure of phase III of solid hydrogen. Phys. Rev. B94, 134101 (2016). ADSGoogle Scholar
  92. Heyd, J., Scuseria, G. E. & Ernzerhof, M. Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys.118, 8207–8215 (2003). ADSGoogle Scholar
  93. Dion, M., Rydberg, H., Schröder, E., Langreth, D. C. & Lundqvist, B. I. Van der Waals density functional for general geometries. Phys. Rev. Lett.92, 246401 (2004). ADSGoogle Scholar
  94. Lee, K., Murray, É. D., Kong, L., Lundqvist, B. I. & Langreth, D. C. Higher-accuracy van der Waals density functional. Phys. Rev. B82, 081101 (2010). ADSGoogle Scholar
  95. Azadi, S. & Ackland, G. J. The role of van der Waals and exchange interactions in high-pressure solid hydrogen. Phys. Chem. Chem. Phys.19, 21829–21839 (2017). Google Scholar
  96. Knudson, M. D., Desjarlais, M. P., Preising, M. & Redmer, R. Evaluation of exchange-correlation functionals with multiple-shock conductivity measurements in hydrogen and deuterium at the molecular-to-atomic transition. Phys. Rev. B98, 174110 (2018). ADSGoogle Scholar
  97. Azadi, S. & Foulkes, W. M. C. Fate of density functional theory in the study of high-pressure solid hydrogen. Phys. Rev. B88, 014115 (2013). ADSGoogle Scholar
  98. Mazzola, G., Helled, R. & Sorella, S. Phase diagram of hydrogen and a hydrogen–helium mixture at planetary conditions by quantum Monte Carlo simulations. Phys. Rev. Lett.120, 025701 (2018). ADSGoogle Scholar
  99. Clay, R. C. et al. Benchmarking exchange-correlation functionals for hydrogen at high pressures using quantum Monte Carlo. Phys. Rev. B89, 184106 (2014). ADSGoogle Scholar
  100. Schöttler, M. & Redmer, R. Ab initio calculation of the miscibility diagram for hydrogen–helium mixtures. Phys. Rev. Lett.120, 115703 (2018). ADSGoogle Scholar
  101. Rillo, G., Morales, M. A., Ceperley, D. M. & Pierleoni, C. Optical properties of high-pressure fluid hydrogen across molecular dissociation. Proc. Natl Acad. Sci. USA116, 9770–9774 (2019). ADSGoogle Scholar
  102. Foulkes, W. M. C., Mitas, L., Needs, R. J. & Rajagopal, G. Quantum Monte Carlo simulations of solids. Rev. Mod. Phys.73, 33–83 (2001). ADSGoogle Scholar
  103. Clay, R. C. III, Holzmann, M., Ceperley, D. M. & Morales, M. A. Benchmarking density functionals for hydrogen–helium mixtures with quantum Monte Carlo: energetics, pressures, and forces. Phys. Rev. B93, 035121 (2016). ADSGoogle Scholar
  104. Chen, J., Ren, X., Li, X.-Z., Alfè, D. & Wang, E. On the room-temperature phase diagram of high pressure hydrogen: an ab initio molecular dynamics perspective and a diffusion Monte Carlo study. J. Chem. Phys.141, 024501 (2014). ADSGoogle Scholar
  105. Azadi, S., Monserrat, B., Foulkes, W. M. C. & Needs, R. J. Dissociation of high-pressure solid molecular hydrogen: a quantum Monte Carlo and anharmonic vibrational study. Phys. Rev. Lett.112, 165501 (2014). ADSGoogle Scholar
  106. Drummond, N. D. et al. Quantum Monte Carlo study of the phase diagram of solid molecular hydrogen at extreme pressures. Nat. Commun.6, 7794 (2015). ADSGoogle Scholar
  107. Azadi, S., Singh, R. & Kühne, T. D. Nuclear quantum effects induce metallization of dense solid molecular hydrogen. J. Comput. Chem.39, 262–268 (2018). Google Scholar
  108. Attaccalite, C. & Sorella, S. Stable liquid hydrogen at high pressure by a novel ab initio molecular-dynamics calculation. Phys. Rev. Lett.100, 114501 (2008). ADSGoogle Scholar
  109. Mazzola, G., Yunoki, S. & Sorella, S. Unexpectedly high pressure for molecular dissociation in liquid hydrogen by electronic simulation. Nat. Commun.5, 3487 (2014). ADSGoogle Scholar
  110. Mazzola, G., Zen, A. & Sorella, S. Finite-temperature electronic simulations without the Born–Oppenheimer constraint. J. Chem. Phys.137, 134112 (2012). ADSGoogle Scholar
  111. Zen, A., Luo, Y., Mazzola, G., Guidoni, L. & Sorella, S. Ab initio molecular dynamics simulation of liquid water by quantum Monte Carlo. J. Chem. Phys.142, 144111 (2015). ADSGoogle Scholar
  112. Mazzola, G. & Sorella, S. Accelerating ab initio molecular dynamics and probing the weak dispersive forces in dense liquid hydrogen. Phys. Rev. Lett.118, 015703 (2017). ADSGoogle Scholar
  113. Delaney, K. T., Pierleoni, C. & Ceperley, D. M. Quantum Monte Carlo simulation of the high-pressure molecular–atomic crossover in fluid hydrogen. Phys. Rev. Lett.97, 235702 (2006). ADSGoogle Scholar
  114. Morales, M. A., Pierleoni, C. & Ceperley, D. M. Equation of state of metallic hydrogen from coupled electron–ion Monte Carlo simulations. Phys. Rev. E81, 021202 (2010). ADSGoogle Scholar
  115. Tubman, N. M., Liberatore, E., Pierleoni, C., Holzmann, M. & Ceperley, D. M. Molecular–atomic transition along the deuterium Hugoniot curve with coupled electron–ion Monte Carlo simulations. Phys. Rev. Lett.115, 045301 (2015). ADSGoogle Scholar
  116. Luo, Y., Zen, A. & Sorella, S. Ab initio molecular dynamics with noisy forces: validating the quantum Monte Carlo approach with benchmark calculations of molecular vibrational properties. J. Chem. Phys.141, 194112 (2014). Google Scholar
  117. Lin, F. et al. Electrical conductivity of high-pressure liquid hydrogen by quantum Monte Carlo methods. Phys. Rev. Lett.103, 256401 (2009). ADSGoogle Scholar
  118. Mazzola, G. & Sorella, S. Distinct metallization and atomization transitions in dense liquid hydrogen. Phys. Rev. Lett.114, 105701 (2015). ADSGoogle Scholar
  119. Zaghoo, M., Husband, R. J. & Silvera, I. F. Striking isotope effect on the metallization phase lines of liquid hydrogen and deuterium. Phys. Rev. B98, 104102 (2018). ADSGoogle Scholar
  120. Davis, P. et al. X-ray scattering measurements of dissociation-induced metallization of dynamically compressed deuterium. Nat. Commun.7, 11189 (2016). ADSGoogle Scholar
  121. McWilliams, R. S., Dalton, D. A., Mahmood, M. F. & Goncharov, A. F. Optical properties of fluid hydrogen at the transition to a conducting state. Phys. Rev. Lett.116, 255501 (2016). ADSGoogle Scholar
  122. Clay, R. C., Desjarlais, M. P. & Shulenburger, L. Deuterium Hugoniot: pitfalls of thermodynamic sampling beyond density functional theory. Phys. Rev. B100, 075103 (2019). ADSGoogle Scholar
  123. Geng, H. Y., Wu, Q., Marqués, M. & Ackland, G. J. Thermodynamic anomalies and three distinct liquid–liquid transitions in warm dense liquid hydrogen. Phys. Rev. B100, 134109 (2019). ADSGoogle Scholar
  124. Holzmann, M. et al. Theory of finite size effects for electronic quantum Monte Carlo calculations of liquids and solids. Phys. Rev. B94, 035126 (2016). ADSGoogle Scholar
  125. Cheng, B., Mazzola, G. & Ceriotti, M. Evidence for supercritical behavior of high-pressure liquid hydrogen. Preprint at http://arxiv.org/abs/1906.03341 (2019).
  126. Soubiran, F. & Militzer, B. Miscibility calculations for water and hydrogen in giant planets. Astrophys. J.806, 228 (2015). ADSGoogle Scholar
  127. Wilson, H. F. & Militzer, B. Rocky core solubility in Jupiter and giant exoplanets. Phys. Rev. Lett.108, 111101 (2012). ADSGoogle Scholar
  128. Ancilotto, F., Chiarotti, G. L., Scandolo, S. & Tosatti, E. Dissociation of methane into hydrocarbons at extreme (planetary) pressure and temperature. Science275, 1288–1290 (1997). ADSGoogle Scholar
  129. Chau, R., Hamel, S. & Nellis, W. J. Chemical processes in the deep interior of Uranus. Nat. Commun.2, 203 (2011). ADSGoogle Scholar
  130. Cytter, Y. et al. Transition to metallization in warm dense helium–hydrogen mixtures using stochastic density functional theory within the Kubo–Greenwood formalism. Phys. Rev. B100, 195101 (2019). ADSGoogle Scholar
  131. Loubeyre, P., Le Toullec, R. & Pinceaux, J. P. Binary phase diagrams of H2–He mixtures at high temperature and high pressure. Phys. Rev. B36, 3723–3730 (1987). ADSGoogle Scholar
  132. Loubeyre, P., Letoullec, R. & Pinceaux, J. A new determination of the binary phase diagram of H2–He mixtures at 296 K. J. Phys. Condens. Matter3, 3183 (1991). ADSGoogle Scholar
  133. Lim, J. & Yoo, C.-S. Phase diagram of dense H2−He mixtures: evidence for strong chemical association, miscibility, and structural change. Phys. Rev. Lett.120, 165301 (2018). ADSGoogle Scholar
  134. Turnbull, R. et al. Reactivity of hydrogen–helium and hydrogen–nitrogen mixtures at high pressures. Phys. Rev. Lett.121, 195702 (2018). ADSGoogle Scholar
  135. Stevenson, D. J. & Salpeter, E. E. The dynamics and helium distribution in hydrogen–helium fluid planets. Astrophys. J. Suppl.35, 239–261 (1977). ADSGoogle Scholar
  136. Stevenson, D. J. & Salpeter, E. E. The phase diagram and transport properties for hydrogen–helium fluid planets. Astrophys. J. Suppl.35, 221–237 (1977). ADSGoogle Scholar
  137. Morales, M. A. et al. Phase separation in hydrogen–helium mixtures at Mbar pressures. Proc. Natl Acad. Sci. USA106, 1324–1329 (2009). ADSGoogle Scholar
  138. Soubiran, F., Mazevet, S., Winisdoerffer, C. & Chabrier, G. Optical signature of hydrogen–helium demixing at extreme density–temperature conditions. Phys. Rev. B87, 165114 (2013). ADSGoogle Scholar
  139. Guillot, T. The interiors of giant planets: models and outstanding questions. Annu. Rev. Earth Planet. Sci.33, 493–530 (2005). ADSGoogle Scholar
  140. Fortney, J. J. et al. in Saturn in the 21st Century (eds. Baines, K.,Flasar, F.,Krupp, N. & Stallard, T.) p. v (Cambridge Univ. Press, 2018).
  141. Militzer, B., Soubiran, F., Wahl, S. M. & Hubbard, W. Understanding Jupiter’s interior. J. Geophys. Res. Planet.121, 1552–1572 (2016). ADSGoogle Scholar
  142. Helled, R. & Guillot, T. Internal Structure of Giant and Icy Planets: Importance of Heavy Elements and Mixing, 44 (Springer, 2018).
  143. Helled, R. The Interiors of Jupiter and Saturn, 175 (Oxford Univ. Press, 2018).
  144. Leconte, J. & Chabrier, G. A new vision of giant planet interiors: impact of double diffusive convection. Astron. Astrophys.540, A20 (2012). ADSGoogle Scholar
  145. Leconte, J. & Chabrier, G. Layered convection as the origin of Saturn’s luminosity anomaly. Nat. Geosci.6, 347–350 (2013). ADSGoogle Scholar
  146. Debras, F. & Chabrier, G. New models of Jupiter in the context of Juno and Galileo. Astrophys. J.872, 100 (2019). ADSGoogle Scholar
  147. Vazan, A., Helled, R. & Guillot, T. Jupiter’s evolution with primordial composition gradients. Astron. Astrophys. 610, L14 (2018).
  148. Marley, M. S., Gómez, P. & Podolak, M. Monte Carlo interior models for Uranus and Neptune. J. Geophys. Res.100, 23349–23354 (1995). ADSGoogle Scholar
  149. Podolak, M., Podolak, J. I. & Marley, M. S. Further investigations of random models of Uranus and Neptune. Planet. Space. Sci.48, 143–151 (2000). ADSGoogle Scholar
  150. Helled, R., Schubert, G. & Anderson, J. D. Empirical models of pressure and density in Saturn’s interior: implications for the helium concentration, its depth dependence, and Saturn’s precession rate. Icarus199, 368–377 (2009). ADSGoogle Scholar
  151. Guillot, T. & Gautier, D. in Treatise on Geophysics. 2nd edn. Volume 10, 529–557 https://www.elsevier.com/books/treatise-on-geophysics/schubert/978-0-444-53802-4 (2015).
  152. Helled, R. et al. in Protostars and Planets VI (eds Beuther, H. et al.) 643 (2014).
  153. Fortney, J. J. & Hubbard, W. B. Phase separation in giant planets: inhomogeneous evolution of Saturn. Icarus164, 228–243 (2003). ADSGoogle Scholar
  154. Mankovich, C., Fortney, J. J. & Moore, K. L. Bayesian evolution models for Jupiter with helium rain and double-diffusive convection. Astrophys. J.832, 113 (2016). ADSGoogle Scholar
  155. Vazan, A., Helled, R., Podolak, M. & Kovetz, A. The evolution and internal structure of Jupiter and Saturn with compositional gradients. Astrophys. J.829, 118 (2016). ADSGoogle Scholar
  156. Püstow, R., Nettelmann, N., Lorenzen, W. & Redmer, R. H/He demixing and the cooling behavior of Saturn. Icarus267, 323–333 (2016). ADSGoogle Scholar
  157. Debras, F. & Chabrier, G. New models of Jupiter in the context of Juno and Galileo. Astrophys. J.872, 100 (2019). ADSGoogle Scholar
  158. Bolton, S. J. et al. Jupiter’s interior and deep atmosphere: the initial pole-to-pole passes with the Juno spacecraft. Science356, 821–825 (2017). ADSGoogle Scholar
  159. Iess, L. et al. Measurement of Jupiter’s asymmetric gravity field. Nature555, 220–222 (2018). ADSGoogle Scholar
  160. Wahl, S. M. et al. Comparing Jupiter interior structure models to Juno gravity measurements and the role of a dilute core. Geophys. Res. Lett.44, 4649–4659 (2017). ADSGoogle Scholar
  161. Nettelmann, N. Low- and high-order gravitational harmonics of rigidly rotating Jupiter. Astron. Astrophys.606, A139 (2017). ADSGoogle Scholar
  162. Guillot, T. et al. A suppression of differential rotation in Jupiter’s deep interior. Nature555, 227–230 (2018). ADSGoogle Scholar
  163. Helled, R. & Stevenson, D. The fuzziness of giant planets’ cores. Astrophys. J. Lett.840, L4 (2017). ADSGoogle Scholar
  164. Iess, L. et al. Measurement and implications of Saturn’s gravity field and ring mass. Science364, aat2965 (2019). ADSGoogle Scholar
  165. Militzer, B., Wahl, S. & Hubbard, W. B. Models of Saturn’s interior constructed with an accelerated concentric Maclaurin spheroid method. Astrophys. J. 879, 78 (2019).
  166. Helled, R. & Guillot, T. Interior models of Saturn: including the uncertainties in shape and rotation. Astrophys. J.767, 113 (2013). ADSGoogle Scholar
  167. Galanti, E. et al. Saturn’s deep atmospheric flows revealed by the Cassini Grand Finale gravity measurements. Geophys. Res. Lett.46, 616–624 (2019). ADSGoogle Scholar
  168. Fuller, J. Saturn ring seismology: evidence for stable stratification in the deep interior of Saturn. Icarus242, 283–296 (2014). ADSGoogle Scholar
  169. Helled, R. The Interiors of Jupiter and Saturn, 175 (Oxford Univ. Press, 2018).
  170. Lühr, H., Wicht, J., Gilder, S. A. & Holschneider, M. Magnetic Fields in the Solar System, Vol. 448 (Springer, 2018).
  171. French, M. et al. Ab initio simulations for material properties along the Jupiter adiabat. Astrophys. J. Suppl.202, 5 (2012). ADSGoogle Scholar
  172. Liu, J., Goldreich, P. M. & Stevenson, D. J. Constraints on deep-seated zonal winds inside Jupiter and Saturn. Icarus196, 653–664 (2008). ADSGoogle Scholar
  173. Cao, H. & Stevenson, D. J. Zonal flow magnetic field interaction in the semi-conducting region of giant planets. Icarus296, 59–72 (2017). ADSGoogle Scholar
  174. Gastine, T., Wicht, J., Duarte, L. D. V., Heimpel, M. & Becker, A. Explaining Jupiter’s magnetic field and equatorial jet dynamics. Geophys. Res. Lett.41, 5410–5419 (2014). ADSGoogle Scholar
  175. Jones, C. A. A dynamo model of Jupiter’s magnetic field. Icarus241, 148–159 (2014). ADSGoogle Scholar
  176. Wicht, J., Gastine, T., Duarte, L. D. V. & Dietrich, W. Dynamo action of the zonal winds in Jupiter. Astron. Astrophys.629, A125 (2019). ADSGoogle Scholar
  177. Duer, K., Galanti, E. & Kaspi, Y. Analysis of Jupiter’s deep jets combining Juno gravity and time-varying magnetic field measurements. Astrophys. J. Lett.879, L22 (2019). ADSGoogle Scholar
  178. Connerney, J. E. P. et al. A new model of Jupiter’s magnetic field from Juno’s first nine orbits. Geophys. Res. Lett.45, 2590–2596 (2018). ADSGoogle Scholar
  179. Moore, K. M. et al. A complex dynamo inferred from the hemispheric dichotomy of Jupiter’s magnetic field. Nature561, 76–78 (2018). ADSGoogle Scholar
  180. Dougherty, M. K. et al. Cassini magnetometer observations during Saturn orbit insertion. Science307, 1266–1270 (2005). ADSGoogle Scholar
  181. Dougherty, M. K. et al. Saturn’s magnetic field from the Cassini Grand Finale orbits. In AGU Fall Meeting Abstracts, Vol. 2017, U22A-02 (2017).
  182. Cao, H. et al. The landscape of Saturn’s internal magnetic field from the Cassini Grand Finale. Icarus344, 113541 (2020). Google Scholar
  183. Cao, H., Russell, C. T., Wicht, J., Christensen, U. R. & Dougherty, M. K. Saturn’s high degree magnetic moments: evidence for a unique planetary dynamo. Icarus221, 388–394 (2012). ADSGoogle Scholar
  184. Drozdov, A., Eremets, M., Troyan, I., Ksenofontov, V. & Shylin, S. Conventional superconductivity at 203 kelvin at high pressures in the sulfur hydride system. Nature525, 73–76 (2015). ADSGoogle Scholar
  185. Liu, H., Naumov, I. I., Hoffmann, R., Ashcroft, N. & Hemley, R. J. Potential high-Tc superconducting lanthanum and yttrium hydrides at high pressure. Proc. Natl Acad. Sci. USA114, 6990–6995 (2017). ADSGoogle Scholar
  186. Nellis, W. J. et al. Equation of state data for molecular hydrogen and deuterium at shock pressures in the range 2–76 GPa (20–760 kbar). J. Chem. Phys.79, 1480–1486 (1983). ADSGoogle Scholar
  187. Holmes, N. C., Ross, M. & Nellis, W. J. Temperature measurements and dissociation of shock-compressed liquid deuterium and hydrogen. Phys. Rev. B52, 15835–15845 (1995). ADSGoogle Scholar
  188. Collins, G. W. et al. Measurements of the equation of state of deuterium at the fluid insulator–metal transition. Science281, 1178 (1998). ADSGoogle Scholar
  189. Belov, S. I. et al. Shock compression of solid deuterium. J. Exp. Theor. Phys. Lett.76, 433–435 (2002). Google Scholar
  190. Boriskov, G. V. et al. Shock-wave compression of solid deuterium at a pressure of 120 GPa. Dokl. Phys.48, 553–555 (2003). ADSGoogle Scholar
  191. Grishechkin, S. K. et al. Experimental measurements of the compressibility, temperature, and light absorption in dense shock-compressed gaseous deuterium. J. Exp. Theor. Phys. Lett.80, 398–404 (2004). Google Scholar
  192. Knudson, M. D. et al. Principal Hugoniot, reverberating wave, and mechanical reshock measurements of liquid deuterium to 400 GPa using plate impact techniques. Phys. Rev. B69, 144209 (2004). ADSGoogle Scholar
  193. Hicks, D. G. et al. Laser-driven single shock compression of fluid deuterium from 45 to 220 GPa. Phys. Rev. B79, 014112 (2009). ADSGoogle Scholar
  194. Loubeyre, P. et al. Extended data set for the equation of state of warm dense hydrogen isotopes. Phys. Rev. B86, 144115 (2012). ADSGoogle Scholar
  195. Miguel, Y., Guillot, T. & Fayon, L. Jupiter internal structure: the effect of different equations of state. Astron. Astrophys.596, A114 (2016). ADSGoogle Scholar

Acknowledgements

The authors thank the anonymous referees for comments that helped to improve the manuscript. The authors also acknowledge support from W. Nellis, F. Soubrian, S. Sorella, D. Stevenson, N. Nettelmann, J. J. Fortney, Y. Miguel, S. Müller, C. Valletta and A. Cumming. R.H. acknowledges support from the Swiss National Science Foundation (SNSF grant 200020_188460) and thanks the members of the Juno science team for discussions. R.R. acknowledges support by the Deutsche Forschungsgemeinschaft via the projects FOR 2440 and SPP 1992.